Let
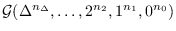
be the set of simple graphs with the degree sequence
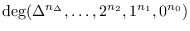
, and
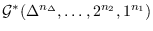
the set of connected graphs with the degree sequence
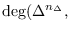
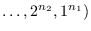
. In this
paper, we first give the numbers of spanning tree of a bicyclic
graph as well as a tricyclic graph, and then show that some degree
sequences of graphs in

(resp.
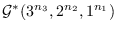
,
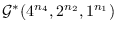
and
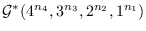
) are
determined by Laplacian spectra (write as
DLS for short) of
the corresponding graphs. Moreover, for the non-
DLS degree
sequences we present some
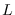
-cospectral mates to indicate that
their
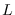
-cospectral degree sequences do exist. By the way, all of
these extend the previous results about Laplacian spectral
determinations of some degree sequences in [23]. Besides, we revise
the references of Theorems 6 and 7 in [23].