Amir Ghasem Ghazanfari, Somayeh Malekinejad: Heron means and Pólya inequality for sector matrices, 329-339

Abstract:

We introduce the Heron means and Pólya inequality for sector matrices and give some inequalities involving them. For instance, we show that if $A,B\in {S_{\alpha}}$ are two sector matrices and $\nu\in[0,1]$, then

$$0\leq F_{\nu}(\mathcal{R}A,\mathcal{R}B)\leq\mathcal{R}F_{\nu}(A,B)\leq \sec^2\alpha F_{\nu}(\mathcal{R}A,\mathcal{R}B)$$

and

$$\cos^3\alpha\Vert H_\nu(A,B)\Vert\leq \Vert F_{\alpha(\nu)}(A,B)\Vert,$$

where $\alpha(\nu)=1-4(\nu-\nu^2)$. We also present the following inequality for the Pólya inequality

$$\left\Vert\int_0^1(A\sharp_{\nu}B)d\nu\right\Vert\leq \sec^3\alpha\left\Vert\frac{2}{3}(A\sharp_{\nu}B)+\frac{1}{3}A\nabla_{\nu}B\right\Vert.$$

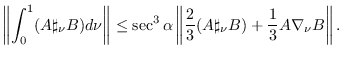Key Words: Sector matrices, Heinz mean, Heron mean.

2010 Mathematics Subject Classification: Primary 15A60; Secondary 15B48.