Let

be any of the following properties: the weak
ideal property, the topological dimension zero,
the combination of pure infiniteness and the
ideal property, residual (SP), pure
infiniteness, strong pure infiniteness, provided that the zero C*-algebra
is included, and
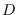
-stability for a separable unital strongly self-absorbing
C*-algebra
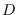
.
Let

be a separable unital C*-algebra with unit
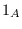
,
and assume that there exist non-zero projections
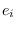
,
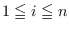
, in

such that
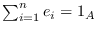
.
We show that

has

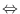
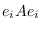
has

for every
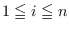
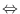

has

(in fact, we prove a much more general
result). We also show that, somehow surprisingly, for two large classes
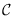
of non-zero C*-algebras, if

,
then the fact that

has the weak ideal property
implies (or, is equivalent to) the fact that

and
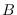
have the weak ideal property. We prove
that one of these two results still holds if we replace the
weak ideal property by some related properties.