In the NFV network, the availability of resource scheduling can be transformed to the existence
of the restricted fractional

-factors in the corresponding NFV network graph. Researching on the
existence of the restricted fractional

-factors in network structure can help to construct the NFV
network with the efficient application of resources. Let
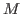
and

be two sets of independent edges of

with

,
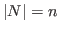
and

. We say that

contains a fractional

-factor
with the property

(or a restricted fractional

-factor) if

has a fractional

-factor

such that
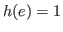
for any
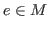
and
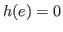
for any
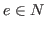
, where
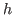
is its indicator function
with
![$h(e)\in[0,1]$](img40.png)
for any
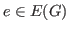
. In this paper, a sufficient condition for graphs to possess restricted
fractional

-factors is presented. Furthermore, it is shown that this result is best possible in some
sense.
-factor.